Notes Dictated to G.E. Moore in Norway: Difference between revisions
No edit summary |
No edit summary |
||
| Line 118: | Line 118: | ||
It follows from the fact that a-b is transitive, that where we have a-b-a the first a has to the second the same relation that it has to b. It is just as from the fact that a-true implies b-false, and b-false implies c-true, we get that a-true implies c-true. And we shall be able to see, having fixed the description of a tautology, that p ≡ ~(~p) is a tautology. | It follows from the fact that a-b is transitive, that where we have a-b-a the first a has to the second the same relation that it has to b. It is just as from the fact that a-true implies b-false, and b-false implies c-true, we get that a-true implies c-true. And we shall be able to see, having fixed the description of a tautology, that p ≡ ~(~p) is a tautology. | ||
That, when a certain rule is given, a symbol is tautological ''shews'' a logical truth.<references /> | That, when a certain rule is given, a symbol is tautological ''shews'' a logical truth. | ||
[[File:Notes Dictated to G.E. Moore in Norway schema.png|300px|center|link=]] | |||
<references /> | |||
Revision as of 18:24, 17 January 2021
Ludwig Wittgenstein
Notes Dictated to G.E. Moore in Norway
Logical so-called propositions show [the] logical properties of language and therefore of [the] Universe, but say nothing. [Cf. 6.12.]
This means that by merely looking at them you can see these proper ties; whereas, in a proposition proper, you cannot see what is true by looking at it. [Cf. 6.113.]
It is impossible to say what these properties are, because in order to do so, you would need a language, which hadn't got the properties in question, and it is impossible that this should be a proper language. Impossible to construct [an] illogical language.
In order that you should have a language which can express or say everything that can be said, this language must have certain properties; and when this is the case, that it has them can no longer be said in that language or any language.
An illogical language would be one in which, e.g., you could put an event into a hole.
Thus a language which can express everything mirrors certain properties of the world by these properties which it must have; and logical so-called propositions shew in a systematic way those properties.
How, usually, logical propositions do shew these properties is this: We give a certain description of a kind of symbol; we find that other symbols, combined in certain ways, yield a symbol of this description; and that they do shews something about these symbols.
As a rule the description [given] in ordinary Logic is the description of a tautology; but others might shew equally well, e.g., a contradiction. [Cf. 6.1202.]
Every real proposition shows something, besides what it says, about the Universe: for, if it has no sense, it can't be used; and if it has a sense, it mirrors some logical property of the Universe.
E.g., take φa, φa ⊃ ψa, ψa. By merely looking at these three, I can see that 3 follows from 1 and 2; i.e. I can see what is called the truth of a logical proposition, namely, of [the] proposition φa . φa ⊃ ψa : ⊃ : ψa. But this is not a proposition; but by seeing that it is a tautology I can see what I already saw by looking at the three propositions: the difference is that I now see THAT it is a tautology. [Cf. 6.1221.]
We want to say, in order to understand [the] above, what properties a symbol must have, in order to be a tautology.
Many ways of saying this are possible:
One way is to give certain symbols; then to give a set of rules for combining them; and then to say: any symbol formed from those symbols, by combining them according to one of the given rules, is a tautology. This obviously says something about the kind of symbol you can get in this way.
This is the actual procedure of [the] old Logic: it gives so-called primitive propositions; so-called rules of deduction; and then says that what you get by applying the rules to the propositions is a logical proposition that you have proved. The truth is, it tells you something about the kind of propositions you have got, viz. that it can be derived from the first symbols by these rules of combination (= is a tautology).
Therefore, if we say one logical proposition follows logically from another, this means something quite different from saying that a real proposition follows logically from another. For so-called proof of a logical proposition does not prove its truth (logical propositions are neither true nor false) but proves that it is a logical proposition (= is a tautology). [Cf. 6.1263.]
Logical propositions are forms of proof: they shew that one or more propositions follow from one (or more). [Cf. 6.1264.]
Logical propositions shew something, because the language in which they are expressed can say everything that can be said.
This same distinction between what can be shewn by the language but not said, explains the difficulty that is felt about types-e.g., as to [the] difference between things, facts, properties, relations. That M is a thing can't be said; it is nonsense: but something is shewn by the symbol "M". In [the] same way, that a proposition is a subject-predicate proposition can't be said: but it is shown by the symbol.
Therefore a THEORY of types is impossible. It tries to say something about the types, when you can only talk about the symbols. But what you say about the symbols is not that this symbol has that type, which would be nonsense for [the] same reason: but you say simply: This is the symbol, to prevent a misunderstanding. E.g., in "aRb", "R" is not a symbol, but that "R" is between one name and another symbolizes. Here we have not said: this symbol is not of this type but of that, but only: This symbolizes and not that. This seems again to make the same mistake, because "symbolizes" is "typically ambiguous". The true analysis is: "R" is no proper name, and, that "R" stands between "a" and "b" expresses a relation. Here are two propositions of different type connected by "and".
It is obvious that, e.g., with a subject-predicate proposition, if it has any sense at all, you see the form, so soon as you understand the proposition, in spite of not knowing whether it is true or false. Even if there were propositions of [the] form "Mis a thing" they would be superfluous (tautologous) because what this tries to say is something which is already seen when you see "M".
In the above expression "aRb", we were talking only of this particular "R", whereas what we want to do is to talk of all similar symbols. We have to say: in any symbol of this form what corresponds to "R" is not a proper name, and the fact that ["R" stands between "a" and "b"] expresses a relation. This is what is sought to be expressed by the nonsensical assertion: Symbols like this are of a certain type. This you can't say, because in order to say it you must first know what the symbol is: and in knowing this you see [the] type and therefore also [the] type of [what is] symbolized. I.e. in knowing what symbolizes, you know all that is to be known; you can't say anything about the symbol.
For instance: Consider the two propositions (1) "What symbolizes here is a thing", (2) "What symbolizes here is a relational fact (= relation)". These are nonsensical for two reasons: (a) because they mention "thing" and "relation"; (b) because they mention them in propositions of the same form. The two propositions must be expressed in entirely different forms, if properly analysed; and neither the word "thing" nor "relation" must occur.
Now we shall see how properly to analyse propositions in which "thing", "relation", etc., occur.
(1) Take φx. We want to explain the meaning of 'In "φx" a thing symbolizes'. The analysis is:—
- (∃y) . y symbolizes . y = "x" . "φx"
["x" is the name of y: "φx" = '"φ" is at [the] left of "x"' and says φx.]
N.B. "x" can't be the name of this actual scratch y, because this isn't a thing: but it can be the name of a thing; and we must understand that what we are doing is to explain what would be meant by saying of an ideal symbol, which did actually consist in one thing's being to the left of another, that in it a thing symbolized.
(N.B. In [the] expression (∃y). φy, one is apt to say this means "There is a thing such that...". But in fact we should say "There is a y, such that..."; the fact that the y symbolizes expressing what we mean.)
In general: When such propositions are analysed, while the words "thing", "fact", etc. will disappear, there will appear instead of them a new symbol, of the same form as the one of which we are speaking; and hence it will be at once obvious that we cannot get the one kind of proposition from the other by substitution.
In our language names are not things: we don't know what they are: all we know is that they are of a different type from relations, etc. etc. The type of a symbol of a relation is partly fixed by [the] type of [a] symbol of [a] thing, since a symbol of [the] latter type must occur in it.
N.B. In any ordinary proposition, e.g., "Moore good", this shews and does not say that "Moore" is to the left of "good"; and here what is shewn can be said by another proposition. But this only applies to that part of what is shewn which is arbitrary. The logical properties which it shews are not arbitrary, and that it has these cannot be said in any proposition.
When we say of a proposition of [the] form "aRb" that what symbolizes is that "R" is between "a" and "b", it must be remembered that in fact the proposition is capable of further analysis because a, R, and b are not simples. But what seems certain is that when we have analysed it we shall in the end come to propositions of the same form in respect of the fact that they do consist in one thing being between two others.
How can we talk of the general form of a proposition, without knowing any unanalysable propositions in which particular names and relations occur? What justifies us in doing this is that though we don't know any unanalysable propositions of this kind, yet we can understand what is meant by a proposition of the form (∃x, y, R) . xRy (which is unanalysable), even though we know no proposition of the form xRy.
If you had any unanalysable proposition in which particular names and relations occurred (and unanalysable proposition = one in which only fundamental symbols = ones not capable of definition, occur) then you can always form from it a proposition of the form (∃x, y, R). xRy, which though it contains no particular names and relations, is unanalysable.
(2) The point can here be brought out as follows. Take φa and φA: and ask what is meant by saying, "There is a thing in φa, and a complex in φA"?
- (1) means: (∃x) . φx . x = a
- (2) means: (∃x, ψξ) . φA = ψx . φx.[1]
Use of logical propositions. You may have one so complicated that you cannot, by looking at it, see that it is a tautology; but you have shewn that it can be derived by certain operations from certain other propositions according to our rule for constructing tautologies; and hence you are enabled to see that one thing follows from another, when you would not have been able to see it otherwise. E.g., if our tautology is of [the] form p ⊃ q you can see that q follows from p; and so on.
Th Bedeutung of a proposition is the fact that corresponds to it, e.g., if our proposition be "aRb", if it's true, the corresponding fact would be the fact aRb, if false, the fact ~aRb. But both "the fact aRb" and "the fact ~aRb" are incomplete symbols, which must be analysed.
That a proposition has a relation (in wide sense) to Reality, other than that of Bedeutung, is shewn by the fact that you can understand it when you don't know the Bedeutung, i.e. don't know whether it is true or false. Let us express this by saying "It has sense" (Sinn).
In analysing Bedeutung, you come upon Sinn as follows: We want to explain the relation of propositions to reality.
The relation is as follows: Its simples have meaning = are names of simples; and its relations have a quite different relation to relations; and these two facts already establish a sort of correspondence between proposition which contains these and only these, and reality: i.e. if all the simples of a proposition are known, we already know that we CAN describe reality by saying that it behaves[2] in a certain way to the whole proposition. (This amounts to saying that we can compare reality with the proposition. In the case of two lines we can compare them in respect of their length without any convention: the comarison is automatic. But in our case the possibility of comparison depends upon the conventions by which we have given meanings to our simples (names and relations).)
It only remains to fix the method of comparison by saying what about our simples is to say what about reality. E.g., suppose we take two lines of unequal length: and say that the fact that the shorter is of the length it is is to mean that the longer is of the length it is. We should then have established a convention as to the meaning of the shorter, of the sort we are now to give.
From this it results that "true" and "false" are not accidental properties of a proposition, such that, when it has meaning, we can say it is also true or false: on the contrary, to have meaning means to be true or false: the being true or false actually constitutes the relation of the proposition to reality, which we mean by saying that it has meaning (Sinn).
There seems at first sight to be a certain ambiguity in what is meant by saying that a proposition is "true", owing to the fact that it seems as if, in the case of different propositions, the way in which they correspond to the facts to which they correspond is quite different. But what is really common to all cases is that they must have the general form of a proposition. In giving the general form of a proposition you are explaining what kind of ways of putting together the symbols of things and relations will correspond to (be analogous to) the things having those relations in reality. In doing thus you are saying what is meant by saying that a proposition is true; and you must do it once for all. To say "This proposition has sense" means '"This proposition is true" means ... .' ("p" is true = "p" . p. Def. : only instead of "p" we must here introduce the general form of a proposition.)[3]
It seems at first sight as if the ab notation must be wrong, because it seems to treat true and false as on exactly the same level. It must be possible to see from the symbols themselves that there is some essential difference between the poles, if the notation is to be right; and it seems as if in fact this was impossible.
The interpretation of a symbolism must not depend upon giving a different interpretation to symbols of the same types.
How asymmetry is introduced is by giving a description of a particular form of symbol which we call a "tautology". The description of the ah-symbol alone is symmetrical with respect to a and b; but this description plus the fact that what satisfies the description of a tautology is a tautology is asymmetrical with regard to them. (To say that a description was symmetrical with regard to two symbols, would mean that we could substitute one for the other, and yet the description remain the same, i.e. mean the same.)
Take p.q and q. When you write p.q in the ab notation, it is impossible to see from the symbol alone that q follows from it, for if you were to interpret the true-pole as the false, the same symbol would stand for p ∨ q, from which q doesn't follow. But the moment you say which symbols are tautologies, it at once becomes possible to see from the fact that they are and the original symbol that q does follow.
Logical propositions, OF COURSE, all shew something different: all of them shew, in the same way, viz. by the fact that they are tautologies, but they are different tautologies and therefore shew each something different.
What is unarbitrary about our symbols is not them, nor the rules we give; but the fact that, having given certain rules, others are fixed = follow logically. [Cf. 3.342.]
Thus, though it would be possible to interpret the form which we take as the form of a tautology as that of a contradiction and vice versa, they are different in logical form because though the apparent form of the symbols is the same, what symbolizes in them is different, and hence what follows about the symbols from the one interpretation will be different from what follows from the other. But the difference between a and b is not one of logical form, so that nothing will follow from this difference alone as to the interpretation of other symbols. Thus, e.g., p.q, p ∨ q seem symbols of exactly the same logical form in the ab notation. Yet they say something entirely different; and, if you ask why, the answer seems to be: In the one case the scratch at the top has the shape b, in the other the shape a. Whereas the interpretation of a tautology as a tautology is an interpretation of a logical form, not the giving of a meaning to a scratch of a particular shape. The important thing is that the interpretation of the form of the symbolism must be fixed by giving an interpretation to its logical properties, not by giving interpretations to particular scratches.
Logical constants can't be made into variables: because in them what symbolizes is not the same; all symbols for which a variable can be substituted symbolize in the same way.
We describe a symbol, and say arbitrarily "A symbol of this description is a tautology". And then, it follows at once, both that any other symbol which answers to the same description is a tautology, and that any symbol which does not isn't. That is, we have arbitrarily fixed that any symbol of that description is to be a tautology; and this being fixed it is no longer arbitrary with regard to any other symbol whether it is a tautology or not.
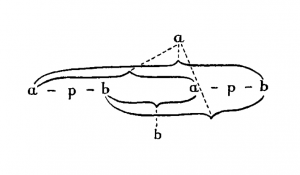
Having thus fixed what is a tautology and what is not, we can then, having fixed arbitrarily again that the relation a-b is transitive get from the two facts together that "p ≡ ~(~p)" is a tautology. For ~(~p) = a-b-a-p-b-a-b. The point is: that the process of reasoning by which we arrive at the result that a-b-a-p-b-a-b is the same symbol as a-p-b, is exactly the same as that by which we discover that its meaning is the same, viz. where we reason if b-a-p-b-a, then not a-p-b, if a-b-a-p-b-a-b then not b-a-p-b-a, therefore if a-b-a-p-b-a-b, then a-p-b.
It follows from the fact that a-b is transitive, that where we have a-b-a the first a has to the second the same relation that it has to b. It is just as from the fact that a-true implies b-false, and b-false implies c-true, we get that a-true implies c-true. And we shall be able to see, having fixed the description of a tautology, that p ≡ ~(~p) is a tautology.
That, when a certain rule is given, a symbol is tautological shews a logical truth.
- ↑ ξ is Frege's mark of an Argumentstelle, to show that ψ is a Funktionsbuchstabe. [Edd.]
- ↑ Presumably "verhält sich zu", i.e. "is related." [Edd.]
- ↑ The reader should remember that according to Wittgenstein '"p"' is not a name but a description of the fact constituting the proposition. See above, p. 109. [Edd.]